Alliance calculatorgeek.com

Simplifying square roots is really easy. You just have to factor the number under the radical, and then pull out any perfect roots from under the radical. To make things easier while simplifying square roots, just memorize a few perfect squares. For example, you can memorize the squares of numbers 1-10.

Still don’t get it? Fear not, we will show you how to simplify radicals in an easy, understandable process.

Step 1: find the Factors that form a perfect square

Take the number under the radical sign and explore which numbers it is evenly divisible by. An even number can always be broken down by 2. For uneven numbers continue to divide by 3, 4, 5 and so on until you hit the correct number.

Still not clear? Let’s give it a practice try.

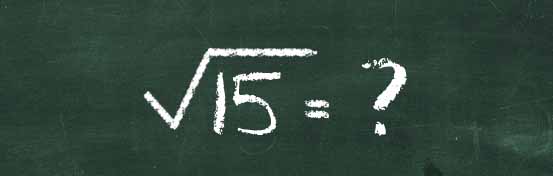
-First we'll find the factors that form this radical √15 by seeking what number 15 is evenly divisible by.

-15 divided by 3 will get you to the even number of 5

-So √15 = √5x3 will be your answer

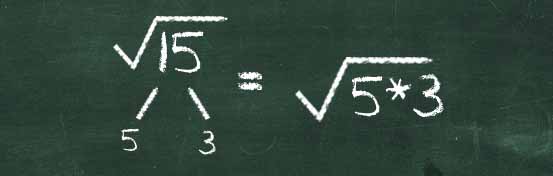
See? Easy as pie!

Step 2: break down the factors to simplest form

If the factors in your answer are not prime numbers lets break them down till they are.

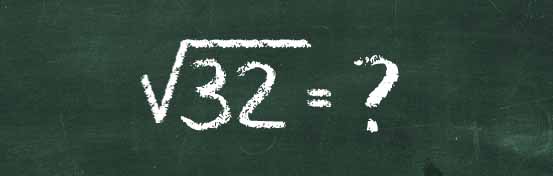
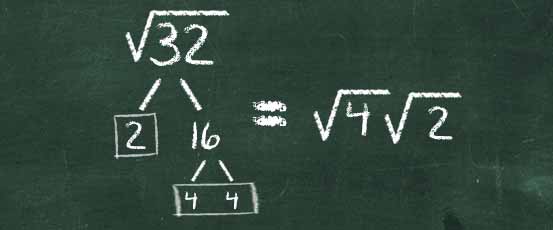
- 32 is a even number so we can divide it by 2 and our answer will be √32 = √16x2

- Give each number in the answer its own radical √32 = √16 √2

- The number 2 is a prime (only divisible by itself or 1) but 16 can still be broken down to 4

- √32 = √4 √2 is your final answer

You have now successfully learnt to simplify square roots!